Economic Multipliers in the EB-5 Arena: Voodoo Economics or Sound Economic Practice? (Vol. 3, Issue 2, July 2015, pgs. 50-55)
By Scott W. Barnhart, PhD, Cora M. Barnhart, PhD and Alan W. Hodges, PhD
Introduction
The pending legislative changes to the current EB-5 law this year, along with the potential renewal of the program, has caused a number of legislators to question the job creation aspects of the program and in particular the definition, interpretation and practicality of regional economic multipliers used in job creation analysis. The Leahy-Grassley bill, S. 1501, for instance, proposes to severely limit use of indirect job creation analysis. There has even been an erroneous suggestion by some that the models and multipliers used for job creation estimates in the EB-5 industry were created solely in response to the advent of the EB-5 program. This article attempts to explain regional economic multipliers, what they are based on, and why the estimated jobs attributed to these multipliers are a “reasonable methodology” as required by the EB-5 law.
Given the abstract nature of the job creation methodology, questions related to the definition and meaning of economic multipliers are certainly understandable. This article examines the origin of macroeconomic multipliers, which date back to the 1930s; and Input-Output models, used in EB-5 job creation studies, which date back even further — to the 1870s. The article also defines macroeconomic and regional economic multipliers, illustrating the use of regional multipliers and their direct, indirect and induced job creation effects within the context of a hypothetical $100 million construction project in the Miami-Fort Lauderdale-West Palm Beach, Florida Metropolitan Statistical Area (MSA).
Simple Macroeconomic Multipliers and the Concept of Multiplier Effects
Both regional and macroeconomic multipliers share common characteristics in that they: a) are based on the concept of multiple and diminishing rounds of spending from an initial change in economic activity; and b) are generally used to determine the effects of an initial change in economic activity (spending or employment) on subsequent changes in national or regional income and employment.
Macroeconomic multipliers were introduced to the public in 1936 by the British economist John Maynard Keynes in his book The General Theory of Employment, Interest and Money. The Keynesian aggregate demand multiplier is an arithmetic expression that shows the change in Gross Domestic Product (GDP), a measure of national income or value added, as a result of the change in one of its major components of consumption, investment or government expenditures, and net exports. The salient point, and why it is called the “multiplier effect,” is that the initial change in, say, investment spending, produces a multiplied effect on the subsequent change in national income that is four to five times larger than the initial change. The process occurs because the initial change in expenditures triggers a chain reaction, causing multiple and diminishing rounds of spending from the increased income as “leakages” occur due to taxes, savings, or spending outside the region. The process is based on the marginal propensity to consume, or MPC, which is the change in consumption expenditures due to an additional $1 of income, which is typically assumed to be about 0.8:

What this means is that for every dollar of income we earn, we spend 80 cents on the consumption of goods and services and save or otherwise dispose of the remaining 20 cents. In turn, that 80 cents represents income for other consumers and businesses in the economy who then spend 80% of it, or 64 cents, and save the other 16 cents. Spending in the next round is 51.2 cents (.8 x 64) and the process continues until all the earnings are exhausted as they are spent, re-spent and saved or taxed in the economy. The concept of the multiple rounds of spending is illustrated in the Figure 1 below.
|
|
| Figure 1. Illustration of the Multiple Rounds of Spending From an Initial $100 Increase in Government Spending |
These multiple rounds of spending based on an MPC=.8 result in a simple macroeconomic multiplier shown in any Principals of Economics text book of 5, which implies that for every $1 dollar increase in investment or government spending, GDP increases by $5.
So if we consider an increase of $100 billion in business investment spending, it will produce a $500 billion increase in Gross Domestic Product:
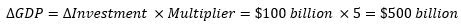
The MPC determines the magnitude of the multiplier. As households spend more of their income on consumption, the MPC increases and the multiplier becomes larger, resulting in larger changes in GDP from initial changes in investment and government spending. For example, if the MPC is .9, the multiplier would be 10 (10=1/(1-.9)). So a change in investment spending of $100 billion would change GDP by $1 trillion.
Input Output Models
Like macroeconomic multipliers, regional Input-Output (IO) model multipliers calculate the total change in value added (Gross Regional Product), labor income, industry output (the value of production or sales of all goods and services in the region), and employment, from an initial change in spending due to, say, construction of a new high-rise condominium in Miami Beach, FL. IO models use detailed data on inter-industry transactions flowing between industry sectors in the economy that produce goods and services (outputs) and these same industry sectors that are purchasing these goods and services (inputs), along with the demand for these goods and services from households, government, and exports.
While the multipliers used in Keynesian analysis date back to the 1930s, IO analysis dates back even further. Although Leon Walras (1874) was probably the first to formally consider an IO framework in the modern sense, Wassily Leontief (1941) is largely credited for developing a matrix model of the American economy that specified how inputs in one industry produce outputs that are either consumed or used as input into another industry. Leontief received a Nobel Prize for this work in 1973. His matrix or table details how an initial change in the production of a final good will initiate changes in the demand for inputs needed to produce that good. Suppose a 10 percent increase in automobile production occurs. We would expect this to result in an increase in steel, labor, machinery, and other resources necessary for the additional output. An input-output table allows an estimation of the additional amounts of these resources needed, including the number of employees in each of these industries. Today, probably the most widely adopted and well-respected IO models are RIMS II created by the U.S. Bureau of Economic Analysis and IMPLAN from the IMPLAN Group, LLC.
IO models are one of the “reasonable methodologies” cited in accordance with 8 C.F.R. § 204.6(j)(4)(iii) and specifically described in 8 C.F.R. § 204.6(m)(3)(v) that can be used to provide evidence of job creation for EB-5 projects. Congress obviously knew about the existence and reliability of IO models when they enacted the Pilot Program for regional centers which was created on October 6, 1992. The Code of Federal Regulations published on August 24, 1993, contained the following language concerning demonstration of job creation: “…supported by economically or statistically valid forecasting tools, including, but not limited to, feasibility studies, analyses of foreign and domestic markets for the goods or services to be exported, and/or multiplier tables.”
Of course, IO analysis and economic multipliers are not only used for EB-5 analysis, they are also used nationally and internationally to estimate the economic impacts of a wide variety of public and private projects including: the impacts from the openings and/or closures of new stadiums, universities, corporate offices, manufacturing plants, federal projects, etc. Similar to the EB-5 industry, where the primary focus in the use of IO models is to estimate the number of jobs, most federal, state, and local authorities who have attracted new industries to their locales, also have a keen interest in new jobs created, but also in household income, new state or local taxes and other new support businesses.
IO Model Multiplier Definitions and Effects
As mentioned above, the multipliers produced by modern IO models estimate the total change in employment, labor income, value added, and output as a result of an initial change in employment or spending (“Final Demand”) in the region for hundreds of distinct industry and governmental sectors. Here we focus on employment multipliers as this is the primary focus in the EB-5 industry. The multipliers are illustrated for a hypothetical $100 million condominium construction project in the south Florida area within the Miami-Fort Lauderdale-West Palm Beach, Florida MSA.
There are two distinct types of multipliers in IO models, and the choice of which to use is based on the inputs the analyst uses to represent the initial change in economic activity being analyzed. The Direct-Effect Employment Multiplier is used if the analyst is using the number of new employees hired or new employee head count for the project as the model input. The Final Demand (or Spending) Employment Multiplier is used if the initial change in economic activity is measured in dollars of expenditures or revenues; this latter model is often referred to as the “expenditure model” in the EB-5 industry. The multipliers are defined as follows:
Direct Effect Employment Multiplier: The total number of jobs created per new direct employee in the project(s).
Final Demand (or Spending) Employment Multiplier: The total number of jobs created per $1 million dollars of final demand (gross revenues or expenditures).
As most projects in the EB-5 industry currently use Final Demand multipliers, we focus on these multipliers here. Table 1 illustrates some of the most commonly used Final Demand multipliers in the EB-5 industry, representing common construction, professional service and business operations sectors obtained from the IMPLAN 2013 model for the South Florida metro area. Each figure in the table represents the total number of jobs created per $1 million expenditure in that industry. For example, the multiplier for IMPLAN sector 60-Construction of new multifamily residential structuresimplies that for every $1 million of expenditures on construction in the Greater Miami area, 22.7 jobs are created. Likewise, for every $1 million of expenditures (or revenues earned), 26.1 jobs are created in IMPLAN sector 499-Hotels and motels, including casino hotels, 38 jobs are created for Elementary and secondary schools, 34 jobs forNursing and community care facilities, etc. Thus, ignoring inflation adjustments for simplicity, the regional multiplier process from a $100 million construction condominium project including all the multiple rounds of spending as discussed above would create 2,268 jobs ($100 million x 22.68 jobs/M$) in the south Florida area.
The multiplier effects shown in Table 1 can be broken down into their component effects: Direct Effects, Indirect Effects, and Induced Effects. These multiplier component effects are defined as follows:
Direct Effects: The change in employment in an industry per $1 million dollars of final demand in that industry.
Indirect Effects: The response by all local industries caused by inter-industry purchasing per $1 million dollars of final demand from the directly affected industries.
Induced Effects: The change in employment resulting from household spending of labor income generated by the direct and indirect effects per $1 million dollars of final demand for a given industry.
For a typical construction project, the Direct Effects are the jobs at the construction site including: construction laborers, carpenters, ready-mix cement workers, steel workers, electricians, HVAC installers, construction managers, etc. In turn the Indirect Effects are the jobs created by the spending of the businesses that supply the services and gear to construct the structure, such as the purchases of tools and equipment, clothing and safety equipment from local retailers, purchases of lumber, concrete, steel rebar, wire and other materials, and payments to truckers for the transportation of the materials, etc. Finally, the Induced Effects are the purchases by households as a result of the income generated in the area from all of the direct and indirect effects. These are the typical everyday purchases made by households on housing, food, gas, clothing, healthcare, household utilities, etc., resulting from income earned by the direct workers at the site and their purchases as well as the income generated from suppliers and inter-industry purchases by suppliers.
Table 2 provides a breakdown of the construction sector used for condominium construction, 60-Construction of new multifamily residential structures, into its Direct, Indirect and Induced effects multipliers. The implication is for every $1 million in expenditures on residential construction in the Miami MSA, 5.3 direct jobs are created at the site, 5.5 indirect jobs are created in the area from inter-industry spending, and another 11.84 induced jobs are created from the spending by households in the area, bringing the total number of jobs created to 22.68. Similar to the total jobs calculation using the Total Effects Final-Demand multiplier, to calculate the total number of direct jobs from the project, one would use the Direct Effects Final Demand multiplier from Table 2 or 530 jobs ($100 x 5.3), likewise for indirect jobs it is 553 jobs ($100 x 5.53), and 1,184 induced jobs ($100 x 11.84).
As a side note, we have also reported what is known as the Direct Effects Multiplier in RIMS terminology, which is the ratio of total jobs to direct jobs that would be used if an employee number or head count were used in the jobs creation estimates. The Final Demand and the Direct Effects Employment Multipliers are related; if one uses the 5.3 direct jobs multiplied by the Direct Effects multiplier of 4.28, one obtains the Total Effects Multiplier, i.e., 5.3 x 4.28 = 22.68.
Analyzing the full hypothetical $100 million project using sector 60-Construction of new multifamily residential structures in the IMPLAN software, one obtains the following summary results for employment, labor income, value added and output, shown in Table 3.
Total employment impacts are estimated at 2,268 jobs, including 530 direct jobs in the construction sector, 554 indirect jobs, and 1,184 induced jobs. In addition, total household or labor income is $114.7 million, value added to the area is $169.8 million, and total output is $330.3 million. In comparison to the Keynesian multiplier discussed above, the implied GDP or value added multiplier for the Miami MSA is 1.7 ($100 million x 1.698=$169.8 million), and that for output is 3.3, both lower than those cited regularly in economic text books.
For this project, the indirect jobs are roughly the same number as the direct jobs, and induced jobs are approximately half of the total jobs estimated. One may wonder, however, if the indirect and induced jobs estimated above actually exist. One need only ask the suppliers of materials and labor to the construction project, “Do you purchase materials from other businesses and retailers in order to run your business?” The answer, of course, is they must or they cannot be in business pouring cement, or installing steel, electrical products and other trade based products. For example, does the local HVAC business buy duct work, vents, filters, and compressor units from distributors in the region? If they do not, they are not in business.
Questions concerning the nature of indirect and induced jobs may be answered by examining a detailed IMPLAN breakdown of the industries with the largest number of indirect and induced jobs created as a result of the hypothetical $100 million project. Table 4 sorts the detailed IMPLAN job results over the 536 industries covered, showing the top twenty industries in terms of indirect jobs. The industries with the highest numbers of indirect employment include a number of retail sectors, indicating that businesses supplying inputs to the construction project buy items from retail outlets, but also real estate, wholesale trade, employment services, truck transportation, architects, ready-mix concrete, etc. For example, 81 of the 554 indirect employees are employed by clothing retailers.
|
|
| Table 4. The Top Twenty Employer Sectors Sorted by Indirect Jobs for a $100 million Construction Project in the Miami-Fort Lauderdale-West Palm Beach, FL MSA |
What about the induced jobs? A total of 1,184 induced jobs are estimated to be created as a result of the direct and indirect spending effects. Think of all of the places a consumer spends his or her income in a given period: housing, healthcare, various retailers, grocery stores and restaurants, local area dry cleaners, drug stores, gas stations, hairdressers, etc. Table 5 sorts the detailed IMPLAN job results showing the top twenty industries in terms of induced jobs. The real estate sector is the industry with the largest number of induced jobs, which makes sense because housing is typically the largest cost in a consumer’s budget, and this sector includes rental housing. Other sectors with the highest numbers of induced employment include local governments, restaurants, hospitals, physicians, retail, education, auto repair, child care, etc.
|
|
| Table 5. The Top Twenty Employer Sectors Sorted by Induced Jobs for a $100 million Construction Project in the Miami-Fort Lauderdale-West Palm Beach, FL MSA |
What about the realism of indirect and induced jobs? Does it make sense that, say, a total of 25 people in the Miami MSA are employed indirectly in sector 449-Architectural, engineering and related services (A&E) as shown in Table 4? It does on two fronts: 1) this sector includes not only architects, but all engineering and related scientific testing and other professional jobs in this sector; 2) although spawned by the $100 million construction project, the total jobs in this sector is generated by $330 million of spending on output, implying that these are jobs created in the region by subcontractors to the direct A&E contractors at the site, but also by other spending on this sector by all the other industries affected by the project.
Conclusions
Regional IO models are used in a wide variety of applications in the economics profession, most outside of the EB-5 arena, and have been in existence in their more modern form since the 1940s. Regional IO models rely on the concept of economic multipliers which date back to the 1930s and are a widely accepted economic tool used for policy analysis. The multipliers obtained from IO models are based on a very detailed, data driven analysis of the trade flows between producers and purchasers in an economic region. They represent a picture of job creation as accurate as possible with currently available economic data and methods. Moreover, Congress recognized IO models as an acceptable methodology when they enacted the legislation authorizing regional centers.














